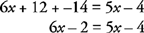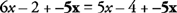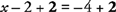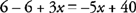Introduction and Section 1, Bourgeois and Proletarians (Part 1)
In earlier ages, we saw society arranged into complicated class structures. For example, in medieval times there were feudal lords, vassals, guild-masters, journeymen, apprentices and serfs. Modern bourgeois society sprouted from the ruins of feudal society. This society has class antagonisms as well, but it is also unique: class antagonisms have become simplified, as society increasingly splits into two rival camps--Bourgeoisie and Proletariat.
The Manifesto then shows how the modern bourgeoisie is the product of several revolutions in the mode of production and of exchange. The development of the bourgeoisie began in the earliest towns, and gained momentum with the Age of Exploration. Feudal guilds couldn't provide for increasing markets, and the manufacturing middle class took its place. However, markets kept growing and demand kept increasing, and manufacture couldn't keep up. This led to the Industrial Revolution. Manufacture was replaced by "Modern Industry," and the industrial middle class was replaced by "industrial millionaires," the modern bourgeois. With these developments, the bourgeoisie have become powerful, and have pushed medieval classes into the background. The development of the bourgeoisie as a class was accompanied by a series of political developments. With the development of Modern Industry and the world-market, the bourgeoisie has gained exclusive political sway. The State serves solely the bourgeoisie's interests.
Historically, the bourgeoisie has played a quite revolutionary role. Whenever it has gained power, it has put to an end all "feudal, patriarchal, idyllic relations." It has eliminated the relationships that bound people to their superiors, and now all remaining relations between men are characterized by self-interest alone. Religious fervor, chivalry and sentimentalism have all been sacrificed. Personal worth is now measured by exchange value, and the only freedom is that of Free Trade. Thus, exploitation that used to be veiled by religious and political "illusions" is now direct, brutal and blatant. The bourgeoisie has changed all occupations into wage-laboring professions, even those that were previously honored, such as that of the doctor. Similarly, family relations have lost their veil of sentimentality and have been reduced to pure money relations.
In the past, industrial classes required the conservation of old modes of production in order to survive. The bourgeoisie are unique in that they cannot continue to exist without revolutionizing the instruments of production. This implies revolutionizing the relations of production, and with it, all of the relations in society. Thus, the unique uncertainties and disturbances of the modern age have forced Man to face his real condition in life, and his true relations with others.
Because the bourgeoisie needs a constantly expanding market, it settles and establishes connections all over the globe. Production and consumption have taken on a cosmopolitan character in every country. This is true both for materials and for intellectual production, as national sovereignty and isolationism becomes less and less possible to sustain. The bourgeoisie draws even the most barbaric nations into civilization and compels all nations to adopt its mode of production. It "creates a world after its own image." All become dependent on the bourgeoisie. It has also increased political centralization.
Thus, we see that the means of production and of exchange, which serve as the basis of the bourgeoisie, originated in feudal society. At a certain stage, however, the feudal relations ceased to be compatible with the developing productive forces. Thus the "fetters" of the feudal system had to be "burst asunder," and they were. Free competition replaced the old system, and the bourgeoisie rose to power.
Marx then says that a similar movement underway at the present moment. Modern bourgeois society is in the process of turning on itself. Modern productive forces are revolting against the modern conditions of production. Commercial crises, due, ironically, to over-production, are threatening the existence of bourgeois society. Productive forces are now fettered by bourgeois society, and these crises represent this tension. Yet in attempting to remedy these crises, the bourgeoisie simply cause new and more extensive crises to emerge, and diminish their ability to prevent future ones. Thus, the weapons by which the bourgeoisie overcame feudalism are now being turned on the bourgeoisie themselves.
Commentary
The Communist Manifesto opens with a statement of its purpose, to publicize the views, aims and tendencies of the Communists. As such it is a document intended to be read by the public, and it is meant to be easily grasped by a general audience. It is also meant to be a broad description of what Communism is, both as a theory and as a political movement.
In this first section, Marx already introduces several of the key ideas of his theory. One main idea is that all of history until now is the story of a series of class struggles. Underlying all of history, then, is this fundamental economic theme. The most important concept being discussed here is the concept that each society has a characteristic economic structure. This structure breeds different classes, which are in conflict as they oppress or are oppressed by each other. However, this situation is not permanent. As history "marches" on, eventually the means of production cease to be compatible with the class structure as-is. Instead, the structure begins to impede the development of productive forces. At this point, the existing structure must be destroyed. This explains the emergence of the bourgeoisie out of feudalism. It will also explain the eventual destruction of the bourgeoisie. Marx believes that all of history should be understood in this way--as the process in which classes realign themselves in compliance with changing means of production.
Perhaps the most significant aspect of this theory of history is what it doesnot deem important. In Marx's theory, history is shaped by economic relations alone. Elements such as religion, culture, ideology, and even the individual human being, play a very little role. Rather, history moves according to impersonal forces, and its general direction is inevitable.
Marx believes that this type of history will not go on forever, however. The Manifesto will later argue that the modern class conflict is the final class conflict; the end of this conflict will mark the end of all class relations. This section begins to suggest why this might be, positing some of the ways in which the modern era is unique. First, class antagonisms have been simplified, as two opposing classes, the bourgeoisie and the proletariat, emerge. Secondly, while exploitative relationships were previously hidden behind things like ideology, now the veil has been lifted and everything is seen in terms of self- interest. Thirdly, in order for the bourgeoisie to continue to exist, they must continually revolutionize the instruments of production. This leaves social relations in an unprecedentedly unstable state...
 =
=  =
= 
 (
(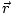 ) =
) = 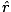 F(r)
F(r)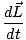 =
= 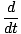 (
(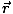 ×
×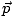 ) =
) = 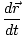 ×
× =
= 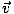 ×(m
×(m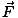 = 0
= 0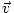
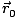
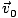
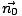 =
= 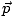
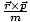 =
=