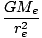Newton and Gravitation
SUMMARY
In 1687 Sir Isaac Newton first published his Philosophiae Naturalis Principia Mathematica (Mathematical Principles of Natural Philosophy) which was a radical treatment of mechanics, establishing the concepts which were to dominate physics for the next two hundred years. Among the book's most important new concepts was Newton's Universal Law of Gravitation. Newton managed to take Kepler's Laws governing the motion of the planets and Galileo's ideas about kinematics and projectile motion and synthesize them into a law which governed both motion on earth and motion in the heavens. This was an achievement of enormous importance for physics; Newton's discoveries meant that the universe was a rational place in which the same principles of nature applied to all objects. The Universal Law of Gravitation has several important features. First, it is an inverse square law, meaning that the strength of the force between two massive objects decreases in proportion to the square of the distance between them as they move farther apart. Second, the direction in which the force acts is always along the line (or vector) connecting the two gravitating objects. Moreover, because there is no "negative mass," gravity is always an attractive force. It is also noteworthy that gravity is a relatively weak force. Modern physicists consider there to be four fundamental forces in nature (the Strong and Weak Nuclear forces, the Electromagnetic force and gravity), of which gravity is the weakest. This means that gravity is only significant when very large masses are being considered.
Terms and Formulae
Terms
Universal Law of Gravitation - Newton's Universal Law of Gravitation states that
where m1 and m2 are the masses of any two objects under consideration and r1 and r2 are their respective position vectors.
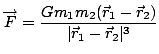 |
where m1 and m2 are the masses of any two objects under consideration and r1 and r2 are their respective position vectors.
Gravitational Constant - This the G that appears as a constant of proportionality in Newton's Universal Law of Gravitation. It has a value of 6.67×10-11 Nm2/kg2.
Formulae
| Equation for the gravitational constant |
|
Newton's Law
Qualitatively Newton's Law of gravitation states that: 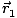 is the position vector of mass m1 and
is the position vector of mass m1 and 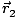 is the position vector of mass m2, then the force on m1 due to m2 is given by:
is the position vector of mass m2, then the force on m1 due to m2 is given by:
The difference of the two vectors in the numerator gives the direction of the force. The appearance of a cube, instead of a square, in the denominator is in order to cancel this direction-giving factor of |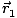 -
- 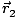 | in the numerator.
| in the numerator. 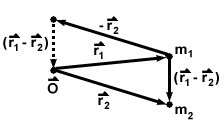
Every massive particle attracts every other massive particle with a force directly proportional to the product of their masses and inversely proportional to the square of distance between them
In vector notation, if 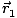 is the position vector of mass m1 and
is the position vector of mass m1 and 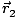 is the position vector of mass m2, then the force on m1 due to m2 is given by:
is the position vector of mass m2, then the force on m1 due to m2 is given by:
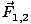 = = 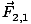 = = 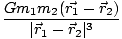 |
The difference of the two vectors in the numerator gives the direction of the force. The appearance of a cube, instead of a square, in the denominator is in order to cancel this direction-giving factor of |
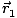 -
- 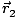 | in the numerator.
| in the numerator. 
Figure 1.1: Direction of force is the difference of the position vectors.
This force has some remarkable properties. First, we note that it acts at a distance , meaning that irrespective of any intervening matter, every particle in the universe exerts a gravitational force on every other particle. Furthermore, gravity obeys a principle of superposition. This means that to find the gravitational force on any particle it is necessary only to find the vector sum of all the forces from all the particles in the system. For example, the force of the earth on the moon is found by vector summing all the forces between all the particles in the moon and earth. This sounds like an immense task, but actually simplifies calculation.
Gravity as a central force
Newton's Universal Law of Gravitation produces a central force. The force is in the radial direction and depends only on the distance between objects. If one of the masses is at the origin, then  (
(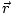 ) =
) = 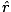 F(r). That is, the force is a function of the distance between the particles and completely in the direction of
F(r). That is, the force is a function of the distance between the particles and completely in the direction of 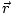 . Obviously, the force is also dependent on G and the masses, but these are just constant--the only coordinate on which the force depends is the radial one.
. Obviously, the force is also dependent on G and the masses, but these are just constant--the only coordinate on which the force depends is the radial one.
 (
(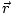 ) =
) = 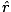 F(r). That is, the force is a function of the distance between the particles and completely in the direction of
F(r). That is, the force is a function of the distance between the particles and completely in the direction of 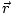 . Obviously, the force is also dependent on G and the masses, but these are just constant--the only coordinate on which the force depends is the radial one.
. Obviously, the force is also dependent on G and the masses, but these are just constant--the only coordinate on which the force depends is the radial one. It is easy to show that when a particle is in a central force, angular momentum is conserved, and motion takes place in a plane. First, let us consider the angular momentum:
The last equality follows because the cross product of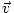 with itself is zero, and since
with itself is zero, and since  is entirely in the direction of
is entirely in the direction of 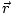 , the cross product of these two vectors is zero also. Since angular momentum does not change over time it is conserved. This is essentially a more general expression of Kepler's Second Law, which we saw (here) also asserted the conservation of angular momentum.
, the cross product of these two vectors is zero also. Since angular momentum does not change over time it is conserved. This is essentially a more general expression of Kepler's Second Law, which we saw (here) also asserted the conservation of angular momentum.
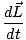 = = 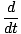 ( (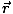 × ×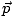 ) = ) = 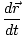 × ×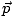 + + 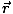 × × = = 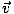 ×(m ×(m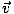 ) + ) + 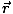 × ×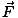 = 0 = 0 |
The last equality follows because the cross product of
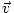 with itself is zero, and since
with itself is zero, and since  is entirely in the direction of
is entirely in the direction of 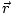 , the cross product of these two vectors is zero also. Since angular momentum does not change over time it is conserved. This is essentially a more general expression of Kepler's Second Law, which we saw (here) also asserted the conservation of angular momentum.
, the cross product of these two vectors is zero also. Since angular momentum does not change over time it is conserved. This is essentially a more general expression of Kepler's Second Law, which we saw (here) also asserted the conservation of angular momentum. At some time t0, we have the position vector 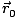 and velocity vector
and velocity vector 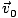 of the motion that define a plane P with a normal given by
of the motion that define a plane P with a normal given by 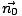 =
= 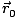 ×
×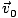 . In the previous proof we showed that
. In the previous proof we showed that 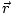 ×
×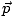 does not change in time. This means that
does not change in time. This means that 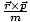 =
= 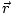 ×
×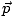 does not change in time either. Therefore,
does not change in time either. Therefore, 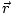 ×
×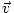 =
=  for all t. Since
for all t. Since 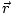 must be orthogonal to
must be orthogonal to  , it must always lie in the plane P.
, it must always lie in the plane P.
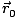 and velocity vector
and velocity vector 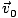 of the motion that define a plane P with a normal given by
of the motion that define a plane P with a normal given by 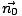 =
= 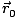 ×
×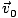 . In the previous proof we showed that
. In the previous proof we showed that 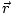 ×
×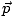 does not change in time. This means that
does not change in time. This means that 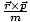 =
= 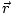 ×
×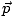 does not change in time either. Therefore,
does not change in time either. Therefore, 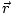 ×
×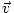 =
=  for all t. Since
for all t. Since 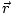 must be orthogonal to
must be orthogonal to  , it must always lie in the plane P.
, it must always lie in the plane P.