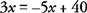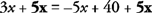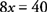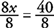Sociologists typically focus their studies on how people and society influence other people, because external, or social, forces shape most personal experiences. These social forces exist in the form of interpersonal relationships among family and friends, as well as among the people encountered in academic, religious, political, economic, and other types of social institutions. In 1959, sociologist C. Wright Mills defined sociological imagination as the ability to see the impact of social forces on individuals' private and public lives. Sociological imagination, then, plays a central role in the sociological perspective.
As an example, consider a depressed individual. You may reasonably assume that a person becomes depressed when something “bad” has happened in his or her life. But you cannot so easily explain depression in all cases. How do you account for depressed people who have not experienced an unpleasant or negative event?
Sociologists look at events from a holistic, or multidimensional, perspective. Using sociological imagination, they examine both personal and social forces when explaining any phenomenon. Another version of this holistic model is the biopsychosocial perspective, which attributes complex sociological phenomena to interacting biological (internal), psychological (internal), and social (external) forces. In the case of depression, chemical imbalances in the brain (biological), negative attitudes (psychological), and an impoverished home environment (social) can all contribute to the problem. The reductionist perspective, which “reduces” complex sociological phenomena to a single “simple” cause, stands in contrast to the holistic perspective. A reductionist may claim that you can treat all cases of depression with medication because all depression comes from chemical imbalances in the brain.
On a topic related to depression, French sociologist Emile Durkheim studied suicide in the late 19th century. Being interested in the differences in rates of suicide across assorted peoples and countries and groups, Durkheim found that social rather than personal influences primarily caused these rates. To explain these differences in rates of suicide, Durkheim examined social integration, or the degree to which people connect to a social group. Interestingly, he found that when social integration is either deficient or excessive, suicide rates tend to be higher. For example, he found that divorced people are more likely to experience poor social integration, and thus are more likely to commit suicide than are married people. As another example, in the past, Hindu widows traditionally committed ritualistic suicide (called “suttee” meaning “good women”) because the cultural pressure at the time to kill themselves overwhelmed them.
Social forces are powerful, and social groups are more than simply the sum of their parts. Social groups have characteristics that come about only when individuals interact. So the sociological perspective and the social imagination help sociologists to explain these social forces and characteristics, as well as to apply their findings to everyday life.